Title: Accelerated Duel: Motorbike vs. Car Dynamics: A motorbike and a car are waiting side by side at traffic lights. When the lights turn to green, the motorbike accelerates at 24 m s2 up to a top speed of….
Introduction:
A motorbike and a car are waiting side by side at traffic lights. When the lights turn to green, the motorbike accelerates at 24 m s2 up to a top speed of…..
The urban landscape transforms into a dynamic arena as a motorbike and a car vie for supremacy at a traffic intersection. When the green light signals the commencement of the race, the motorbike accelerates at a blistering 24 m/s², reaching a formidable top speed of 20 m/s, while the car, with a more measured pace, accelerates at 1 m/s² until it attains a top speed of 30 m/s. This numerical exploration unravels the thrilling dynamics of their acceleration, subsequent constant-speed journey, and the intriguing interplay of time, velocity, and displacement.
Scenario Overview: The narrative unfolds in the heart of a cityscape, capturing the transient acceleration phase and the sustained constant-speed motion of the motorbike and car.
Objectives:
- Velocity Dynamics Display (a): Illustrate the (t, v) graphs for both the motorbike and the car, showcasing the time evolution of their velocities.
- Displacement Drama (b): Sketch the (t, s) graphs to portray the distance covered by each vehicle over time.
- Spatial Reckoning (c): Determine the moment when the motorbike and the car, having pursued their individual trajectories, once again find themselves side by side.
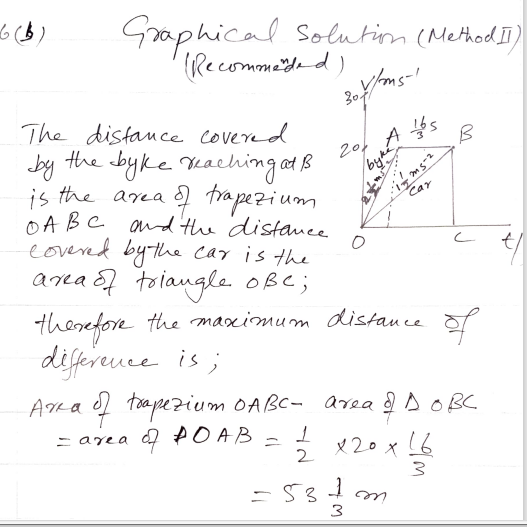
- Distance Duel (d): Uncover the maximum separation distance between the motorbike and the car during this vehicular duel.
Significance: This exploration is significant in unraveling the dynamics of accelerated motion, showcasing the contrasting strategies of the motorbike and the car, and culminating in a spatial rendezvous.
Exploration Focus: The primary focus lies in visualizing and understanding the (t, v) and (t, s) graphs, enabling a comprehensive comprehension of the acceleration, constant-speed, and spatial dynamics.
Dynamics of Acceleration: The exploration navigates through the intricacies of acceleration, constant-speed motion, and the spatial interplay between the motorbike and the car.
Mathematical Calculations: Equations of motion, velocity-time relationships, and displacement calculations guide the mathematical expressions shaping the graphical representations.
Visualization: Graphical representations enhance the narrative, offering a visual spectacle of the motorbike and car dueling through time and space.
Douglas Quadling Mechanics1 Exercise1D Q6

Douglas Quadling Mechanics1 Exercise1D Q6
A motorbike and a car are waiting side by side at traffic lights. When the lights turn to green, the motorbike accelerates at 24 m s2 up to a top speed of……..

Douglas Quadling Mechanics1 Exercise1D Q6
Conclusion:
A motorbike and a car are waiting side by side at traffic lights. When the lights turn to green, the motorbike accelerates at 24 m s2 up to a top speed of……
As the numerical journey unfolds, the exploration promises to capture the essence of this accelerated duel, showcasing the evolving velocities, distance covered, and the thrilling moment when these vehicles, having followed distinct trajectories, converge once again. The quest provides a cinematic glimpse into the dynamic rivalry between the motorbike and the car, exploring the realms of acceleration, constant-speed motion, and spatial dynamics
The Next Question: https://alevelmechanics1.com/582/a-roller-skater-increases-speed-from-4-m-s-to-10-ms-in-10-sec/
