Title: Traffic Dilemma: Acceleration and Deceleration Choices
Introduction: In the urban rhythm, a car approaches a pedestrian crossing with the traffic light transitioning from green to amber. This scenario unfolds a critical decision for the driver: accelerate to clear the crossing before the light turns red or decelerate to a halt at the amber signal. This numerical exploration navigates the physics behind these choices, delving into the least acceleration needed for a timely crossing and the least deceleration required to make a safe stop.
Scenario Overview: Imagine a driver, 25 meters away from a pedestrian crossing, facing the challenge posed by the changing traffic light. The light shifts from green to amber, signaling a pivotal moment for the driver to decide on the course of action.
Objectives: This exploration sets out to uncover the minimum acceleration imperative for the car to safely traverse the crossing before the light turns red. Simultaneously, it examines the least deceleration required for the car to come to a complete stop at the amber signal.
Significance: Understanding the minimum acceleration and deceleration thresholds holds practical significance, contributing to traffic safety considerations and driver decision-making processes.
Acceleration Analysis: In the pursuit of swift traversal, the numerical investigation focuses on identifying the minimum acceleration needed for the car to clear the pedestrian crossing before the onset of the red light.
Deceleration Dilemma: On the contrary, if the driver opts for caution and chooses to stop at the amber signal, the analysis extends to ascertain the minimum deceleration essential for a safe and controlled halt.
Mathematical Calculations: The realms of physics come into play, invoking the equations of motion to calculate the minimum acceleration and deceleration, considering the distance, initial velocity, and time constraints.
Decision Dynamics: This exploration illuminates the delicate balance between acceleration and deceleration decisions, offering insights into the physics guiding responsible driving practices at traffic junctions.
Douglas Quadling Mechanics 1
Exercise 1C Q10
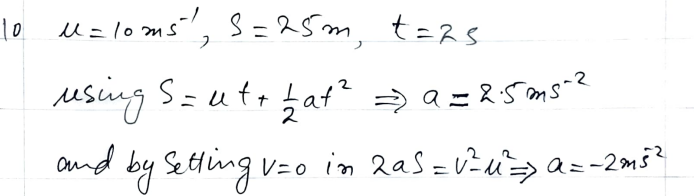
Conclusion:
As the car approaches the pedestrian crossing amidst the changing traffic light dynamics, this numerical exploration quantifies the least acceleration for a timely traverse and the least deceleration for a secure stop. The findings contribute to a deeper understanding of the physics influencing driver decisions at critical moments in urban traffic scenarios.
